8. To calculate the gear ratio, count the number of teeth on gear J and divide it by the number of teeth on gear A. Write down the result.  Then divide the number of teeth on gear E by the number on gear F. Now multiply the two figures together to get the ratio for first gear, or the number of times the clutch must turn to make the drive sprocket turn once.

9. The foot shift lever is pulled up to engage second gear.  Gear I moves to the right, disengaging from gear J. Gear C moves to the left, engaging gear B (see Figure 14).  Gear C's opposing gear, gear H, is not locked to the lay shaft.  Power flows from gear C to gear B to gear i, which is locked to the shaft.  This turns gear F, which turns gear E and the drive sprocket.

10. To calculate the gear ratio, divide the umber of teeth on gear I by the number on gear B. Divide the number on gear E by the number on gear F. Then multiply the two together to get the second gear ratio.

11. The foot shift lever is pulled up to engage third gear.  Gear C disengages from gear B. Gear I engages gear H (see Figure 15).  Power flows to gear C, which is locked to the main shaft.  It's opposing gear, H, is not locked to the lay shaft, but it is engaged to 1, which is.  Gear F turns gear E and the drive sprocket.

12. To calculate the gear ratio, divide the number of teeth on gear H by the number on gear C. Divide the number on gear E by the number on gear F. Multiply the two together to get the third gear ratio.

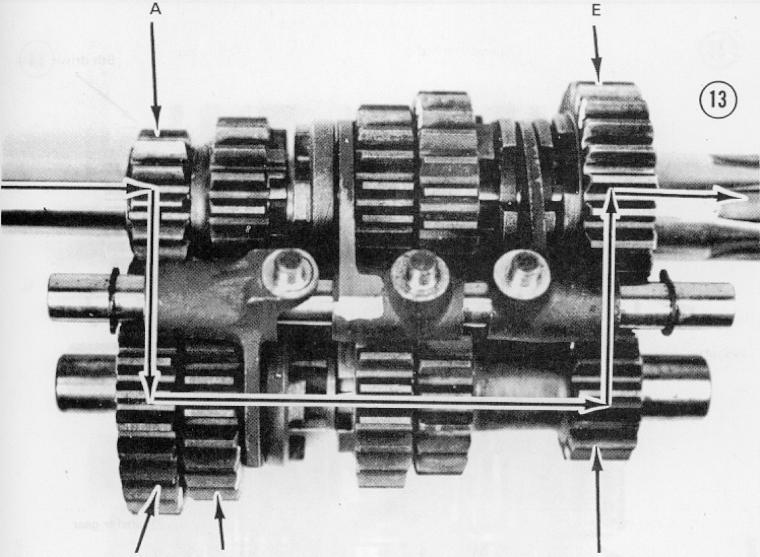
13. The foot shift lever is pulled up to engage fourth gear.  Gear I disengages from gear H. The sliding dog, K, engages gear D (see Figure 16).  Power flows to gear K, which is locked to the main shaft, and to gear D, which is not.  Gear D turns gear G, which is locked to the lay shaft, turning gear F and gear E, along with the drive sprocket.